Top How To Draw Hasse Diagram Of A Poset of all time Check it out now

Diagram hasse poset draw

Table of Contents

If you are a math student, you must have come across Hasse diagrams. A Hasse diagram is a mathematical tool used to represent a finite partially ordered set, in which the relation between elements is drawn in the form of directed acyclic graphs. The diagram presents the poset in a compact and intuitive form.

Understanding Hasse diagrams can be difficult, especially for beginners. Most students find it challenging to draw one from scratch. The process can be frustrating, time-consuming, and requires a lot of effort. It is essential to understand the steps involved in creating a Hasse diagram of a poset to make the process smoother.

Creating a Hasse diagram is not as complicated as it may seem. The first step is to determine the poset’s elements and the relationships between them. Afterward, arrange the elements in a line and draw a line segment that connects the elements with ordered pairs.

In conclusion, creating a Hasse diagram for a poset can be made simpler by following the right steps. Understanding the concept of Hasse diagrams, the elements involved, and the relationship between them are fundamental in creating a comprehensive diagram. With practice and experience, the process becomes more manageable, and you’ll be creating diagrams in no time.

How to draw Hasse diagram of a poset

Hasse diagrams are used to visually represent a finite partially ordered set. The diagrams are drawn in the form of directed acyclic graphs, making it easier to understand the set and its relationships. Here is a step-by-step guide to drawing a Hasse diagram of a poset.

First, list all the items in the poset. Arrange these items in a line from the smallest to the largest element. Draw a line segment connecting any two elements in the poset with an ordered pair. Use arrows pointing upwards to denote the ordering of the elements.

It is essential to note that a Hasse diagram should present elements in a way that ensures no lines cross, and there are no cycles in the graph. To get a clear and concise Hasse diagram, eliminate any unnecessary representation, such as symmetrical pairs.

Tools you might need

Creating a Hasse diagram requires minimal resources. All you need is a pen, paper, and a ruler to make sure that the elements are aligned. The Hasse diagram can be created digitally using drawing software such as Edraw, TikZ, or even online tools like draw.io or Visual Paradigm.

### Understanding the components of Hasse diagram

A Hasse diagram consists of nodes and edges, each with specific functions. The nodes represent the elements in the poset, and edges connect these elements, showing the relationships among them. The edges in Hasse diagrams are represented by arrows pointing upwards from smaller to larger elements.

Common mistakes when drawing Hasse diagrams

There are several common errors to avoid when drawing Hasse diagrams. For instance, representing a relation that does not exist in the poset, such as drawing a line between unrelated elements. Drawing unnatural cycles in the Hasse diagram or not reflecting the partial order in the graph is a mistake that should also be avoided.

How do Hasse diagrams benefit learners?

Hasse diagrams are an essential tool in mathematics, especially in areas that involve set theory and topology. The diagrams help learners understand the relations between elements in a poset without going through the entire set of relations. The Hasse diagrams are visually appealing, and the relationships among elements can be quickly grasped with ease compared to other forms of representation.

Question and Answer

Q: What is a poset?

A: A poset is a set with a partial order relation. The relation must be reflexive, antisymmetric, and transitive for it to be a poset.

Q: Can Hasse diagrams represent infinite posets?

A: No, Hasse diagrams can only represent finite posets.

Q: Can Hasse diagrams be used in computer science?

A: Yes, Hasse diagrams can be used in computer science in various applications such as lattice theory, data mining, database design, and computer networks.

Q: What is the difference between Hasse diagrams and Ven diagram?

A: Hasse diagrams and Ven diagrams are used in mathematics to represent different types of sets. A Ven diagram shows the relation between sets by overlapping circles representing inclusion, whereas a Hasse diagram represents the relation between elements in a poset using a directed acyclic graph.

Conclusion of how to draw Hasse diagram of a poset

In conclusion, Hasse diagrams are an essential tool in mathematics that help learners comprehend the relations between elements in a poset. Drawing Hasse diagrams requires correctly understanding the concept of Hasse diagrams, the elements involved, and the relationship between them. Once the fundamentals are clear, creating Hasse diagrams become effortless. As a mathematical tool, Hasse diagrams are used in various ways, including lattice theory, data mining, database design, and computer networks.

Gallery

[SOLVED] How To Draw A Poset Hasse Diagram Using TikZ? ~ TeX - LaTeX

_{^{Photo Credit by: bing.com /}}

Draw The Hasse Diagram For The Poset (P(U), ), | Chegg.com

_{^{Photo Credit by: bing.com / diagram hasse poset draw}}

How To Draw Hasse Diagram - Free Wiring Diagram

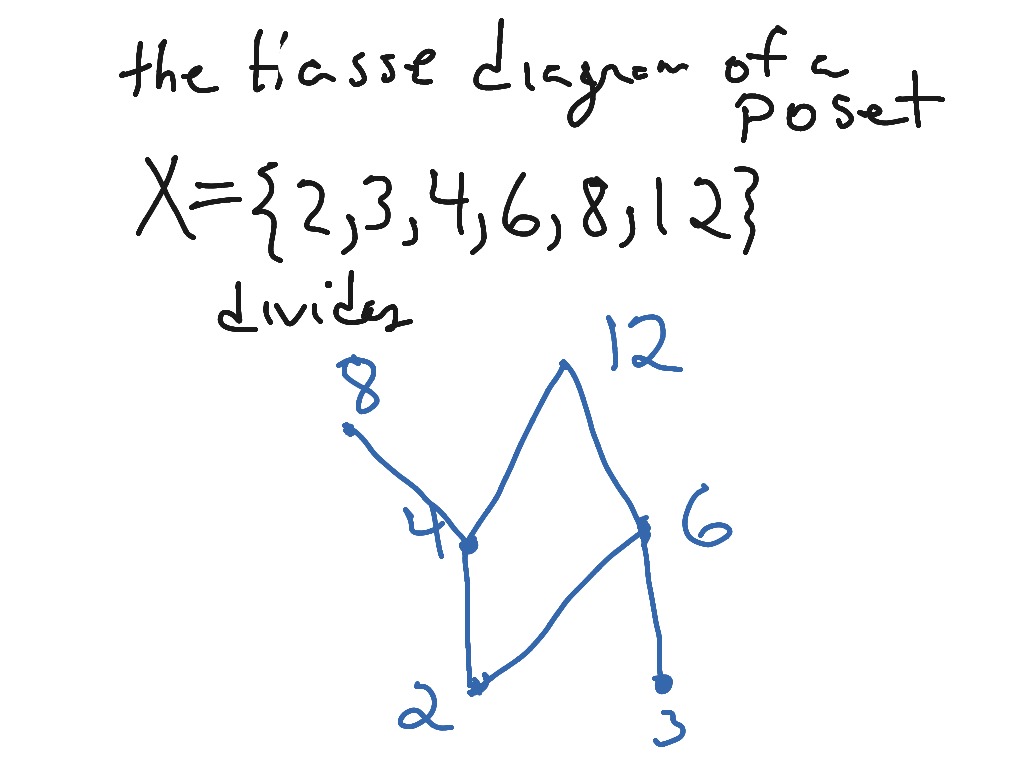
_{^{Photo Credit by: bing.com / hasse diagram draw showme poset}}

Math Diagram - Types, How To & Examples | Edraw

_{^{Photo Credit by: bing.com / hasse poset posets disconnected}}

How To Draw Hasse Diagram - Free Wiring Diagram

_{^{Photo Credit by: bing.com / hasse diagram draw poset using latex tikz stack tex}}